Benchmarking Variational Quantum Eigensolvers for the Kitaev Model
Advancing variational quantum algorithms on NISQ devices
The Science:
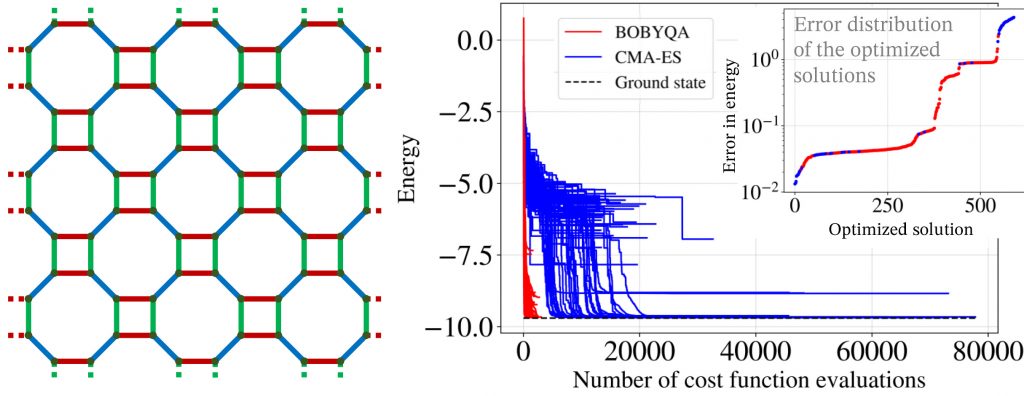
SQMS researchers investigated the optimal strategy to scale up variational quantum eigensolvers into the quantum advantage regime by comparing different optimizers and variational circuit ansatz. They also demonstrated an implementation on current Rigetti Computing hardware using multiple error mitigation techniques.
The Impact:
The benchmark result illustrates how to advance variational quantum algorithms to simulate lattice models into the beyond-classical limit on NISQ devices. The classical simulation reveals the best optimization strategy and variational ansatz for the Kitaev system and the potential of probing the system’s phase diagram using variational algorithms. The NISQ implementation informs about necessary improvements for future device calculations.
Summary:
The frustrated Kitaev spin model on the square-octagon lattice is an ideal benchmark system for Rigetti hardware as it directly maps to the native qubit geometry. We benchmark several optimizers and variational circuit ansatz for calculating the ground state of the square-octagon-lattice Kitaev model using variational quantum eigensolvers. The benchmark result shows that, for larger systems, an optimization strategy involving multiple classical optimizers of different properties is suitable for variational algorithms, and the Hamiltonian variational ansatz is easier to optimize than hardware efficient ansatz. We also show that the phase transition of the frustrated Kitaev spin model can be studied by determining the expectation values and energy gaps using variational quantum eigensolvers. The NISQ implementation of pre-optimized Hamiltonian variational Ansatz circuits shows the need for specific error mitigation techniques.
Contact:
Andy C. Y. Li – cli@fnal.gov
Focus Area:
Quantum Algorithms, Simulation and Benchmarking
Institutions:
Fermilab, Rigetti Computing, Ames National Laboratory, Lockheed Martin, NASA Ames
Citation:
A. C. Y. Li, M. S. Alam, T. Iadecola, A. Jahin, J. Job, D. M. Kurkcuoglu, R. Li, P. P. Orth, A. B. Özgüler, G. N. Perdue, and N. M. Tubman, Benchmarking variational quantum eigensolvers for the square-octagon-lattice Kitaev model, Phys. Rev. Res. 5, 033071 (2023)
Funding Acknowledgements:
This material is based upon work supported by the U.S. Department of Energy, Office of Science, National Quantum Information Science Research Centers, Superconducting Quantum Materials and Systems Center (SQMS) under contract number DE-AC02-07CH11359. Some calculations were performed as part of the XSEDE computational Project No. TG-MCA93S030 on Bridges-2 at the Pittsburgh supercomputer center.
